
Two small charged spheres are 6.52 cm apart. They are moved, and the force each exerts on the other is found to have tripled. How far apart are they now?
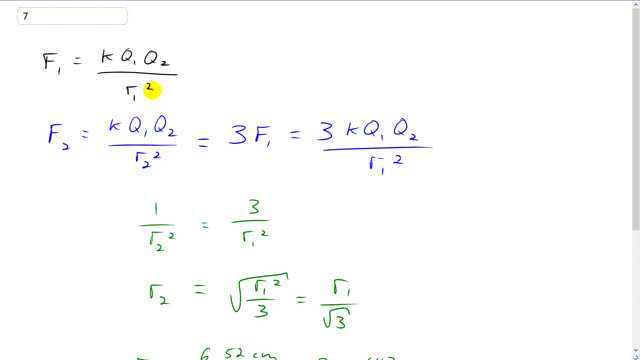
In order to watch this solution you need to have a subscription.
This is Giancoli Answers with Mr. Dychko. The force in the first case his case K Q one Q two over r one squared. So Coulombs constant times the charge in one sphere times to charge in other sphere divided by the distance between them squared and this distance has a subscript one because that's the distance in this first case then in the second case the numerator is the same but the denominator is r two squared and we know that this force is three times what the force was before and three times the force before is three times K Q one Q two over r one squared substituting it for F one here and that means this equals this and the K Q one Q two cancels on both sides and we are left with one over r two squared equals three over r one squared and then take the square root of both sides and flip both sides which is the same as raising both sides to the exponent negative one half the negative exponent flips a fraction or takes the reciprocal in other words and then the making it one half is the same as square rooting. So we have a r two just on its own in the left side. So we flipped end square root of r two is there and on the right hand side it's the square root of r one squared over three which is the same as r one over root three. So the distance in the second case then with when the force is three times what it was before is the original distance divided by root three and that's 6.52 centimeters over root three which is about 3.76 centimeters is the new distance.