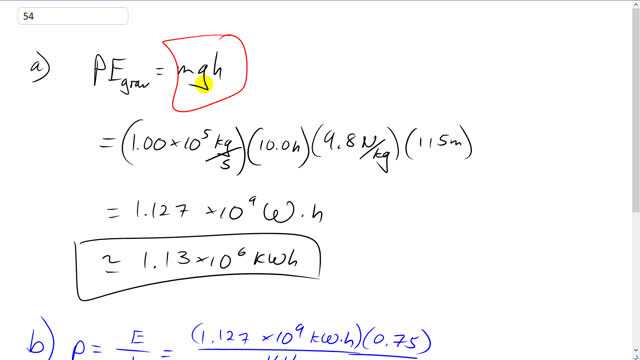
Energy may be stored by pumping water to a high reservoir when demand is low and then releasing it to drive turbines during peak demand. Suppose water is pumped to a lake 115 m above the turbines at a rate of $1.00 \times 10^5 \textrm{ kg/s}$ for 10.0 h at night.

VIDEO TRANSCRIPT

This is Giancoli answers with Mr. Dychko. Assuming the water pump has perfect efficiency, the amount of energy it'll take to pump all this water up during the night will be its gravitational potential energy, mass times acceleration due to gravity g times the height h. So the pump pumps at one times ten to the five kilograms per second and is going to do that for ten hours at night and uh and at times by 9.8 Newtons per kilogram times 115 meters and our units are a bit strange here, we have kilogram per second multiply by ten hours but this is intentional because what we end up with is, is the kilograms cancelling and uh and we have Newtons times meters which make joules divided by second makes joules per second which is Watts and then times by this hour which is what hours and energies and utility companies are typically written in kilowatt hours so take away three from that exponent and you know, you know… divide this by a 1000 and then you can put a kilo prefix there because kilo means times by a 1000. So we have 1.13 times ten to the six kilowatt hours of energy stored. And then if all of this energy is released within 14 hours with an efficiency of 75 percent it can produce power at a rate of 60 megawatts because its the 1.127 times ten to the nine kilowatt hours stored divided by 14 hours and the hours cancel nicely and times by 0.75 efficiency, 60 megawatts.