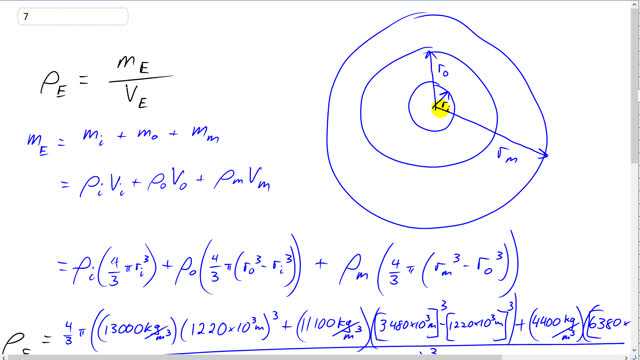
The Earth is not a uniform sphere, but has regions of varying density. Consider a simple model of the Earth divided into three regions—inner core, outer core, and mantle. Each region is taken to have a unique constant density (the average density of that region in the real Earth):

VIDEO TRANSCRIPT

This is Giancoli Answers with Mr. Dychko. The density of the Earth is the Earth's total mass divided by its total volume. Now its total mass with this model of it consisting of three layers is gonna be the mass of the inner core plus the mass of the outer core and then plus the mass of the mantle. And mass of the inner core will be the inner core's density times the inner core volume; mass of the other core will be its density times its volume. And when we calculate this outer core volume, we are gonna be taking the volume of this sphere of radius r subscript o for outer core and subtracting away the volume of this sphere of the inner core. So we'll just be taking the volume of the thick shell and then same for the mantle: when we find the mantle volume, we'll take the volume of the sphere with radius r mantle, r m, and then subtracting away the volume of this sphere here with radius r o for outer core. And when we take the difference between these volumes, it's four-thirds πr m cubed minus four-thirds πr o cubed and this four-thirds π is a common factor we can just factor out and it turns out that you can factor it out of every single thing here so on this line here, I wrote four-thirds π times all of it which wasn't even necessary because on the bottom, it turns out the total volume of the Earth has a four-thirds π factor in it as well so these four-third's π would cancel anyway but I have already punched it into my calculator with four-third's on the top so let's keep it on the bottom too. Now what else can I say? Well, we have some unit issues because the radii we are given are in units of kilometers so we always have to write in times 10 to the 3 meters and then cube it. So we have the inner core density— 13000 kilograms per cubic meter— times the inner core volume. So we are times'ing this by the volume but if you can get the idea that we have this four-third's π factored out and we are just multiplying by the inner core radius here cubed. So that's 1220 times 10 to the 3 meters cubed plus the density of the outer core times the difference in the cubes of the radii between outer core and inner core and on the calculator, it looks like this when you have this capital E notation it's always a good thing to do scientific notation with this capital E at second function comma here because when you go to the power of 3 that exponent 3 is applied intelligently to everything here this entire number considers this 1220E3 as a single number as it should—that's your intention— whereas if you typed in 1220 with a multiply sign and then 10 to the power of 3 and then did exponent 3 that exponent 3 would apply only to a part of the number. So for example, if you did 1220 times 10 to the power of 3 and then go cubed, this would be bad; it would actually be 1220 times 10 to the power of 9 or 10 to the power of 27 is what it would turn out to because because 3 cubed is 27 so yeah that's bad. Always use this capital E notation for scientific notation because then it groups the two parts: the times 10 to the part and the other part together as a single number. Okay! Other than that, everything else is just plug and chug punching in numbers here to get our answer and our answer is 550 kilograms per cubic meter. So if you consider the Earth to have only 1 layer and we take its total mass divided by its total volume what we end up with here is 5.98 times 10 to 3 kilograms and then divide that by four-third's π times 6380 times 10 to the 3 cubed and you end up with the density of... (woah, something went wrong there) that 5.98 times 10 to the 24 that's the problem 24, getting all these cubes on the mind here so many cubes everywhere I wrote a cube there instead. Okay so let's try that again. We go second function enter to get the last entry and then over here, we can insert the 24; everything else looks good. That's more realistic; we expected something close to the 5500 we had before. So that's 5497.3 kilograms per cubic meter. And then the percent difference will be what we just found— 5497.3 kilograms per cubic meter— minus the 5500 from before and that's the absolute difference in the numerator and then divide by the value we are comparing against and we end up with a very small number that's about... well, as a percent so we have to multiply by 100 to get percent here that's negative 0.05 percent off of the density calculated with a model with three layers.